Hexominos Worksheet

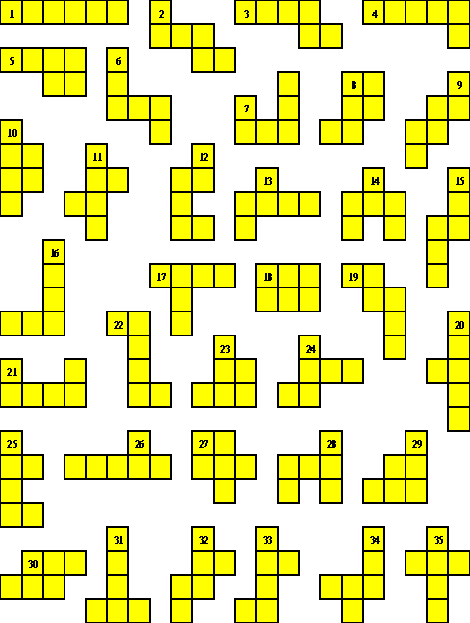
Definition

Hexominos are figures with six squares joining together. Each square has at least one side touching another squares. There are 35 hexominos, omitting the mirror images or rotations of the figures.

35 Hexominos

If you need a printable form of the following worksheet, click here. The file is in pdf.

Search….

1. Which of the above hexominos can fold to form a cube? There are 11 of them.

2. 15 of the 35 hexominos are symmetric.

(a) Find out 5 which are symmetric about the center of the figure.

(b) Find out 2 which are symmetric about 2 axes (and about the center).

(c) Find out eight which are symmetric about 1 axis only.

3. You can classify the 35 hexominos into six groups. Assuming the squares of hexominos
are of sides 1. Which of the hexominos can be placed inside a rectangle of the following
dimensions? (You can rotate the hexominos. The numbers are shown inside the brackets)

(a) 2 x 4 (6)

(b) 3 x 3 (7)

(c) 3 x 4 (15)

(d) 2 x 5 (5)

(e) 1 x 6 (1)

(f) 2 x 3 (1)

4. Colour your figures alternately with two different colours, say red and green, so that the adjacent squares must be of different colour. Find out the hexominos with 2 red and 4 green squares. (or 4 red and 2 green) These are even hexominos. The others, with 3 red and 3 green squares are called odd hexominos.

Answers

Use your mouse to highlight the table to get the answers.

1	2, 9, 11, 13, 15, 22, 24, 31, 32, 33, 35
2 (a)	9, 11, 22, 15, 30
2 (b)	1, 18
2 (c)	10, 14, 20, 21, 27, 29, 31, 35
3(a)	5, 10, 12, 21, 25, 30
3(b)	7, 8, 14, 23, 27, 28, 29
3(c)	2, 6, 9, 11, 13, 16, 17, 19, 22, 24, 31, 32, 22, 34, 35
3(d)	3, 4, 15, 20, 26
3(e)	1
3(f)	18
4	25 - 35