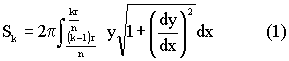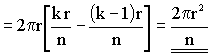An example in Surface Area |
|
|
A fun fact A sphere is cut
on the surface into rings as in the diagram. If the vertical height of each
of the rings remains the same, say k units. Believe it or not - the surface
area of any one of the rings remains constant. |
|
|
Let x2 + y2
= r2 be the circle as in the diagram. We concentrate on the
positive-x part of the function. The [0, r] on the x-axis is cut into n equal
intervals. (Here are 5.):
The width of the ring is
|
|
|
The surface area of the k-th ring, formed by rotating Ik
around x-axis, is given by: Now, x2 + y2 = r2
, therefore Substitute (2) in (1), we
can get: Here Sk is
independent of k (there is no k in the result). Therefore Sk is a
constant. |
|
|
As
there are n rings in positive x direction and n rings in the negative x
direction. The total surface area of
all rings is |
|