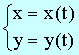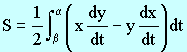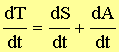A simple way to show a formula in area
Area in parametric form Let C be a parametric curve given by : If the point P(x,y), as t varies from a to b, encircles a loop, the area is :
This formula is rather difficult to understand, here is a simple way to show the result. Referring to the diagram, if T denotes the signed area of the triangle in yellow, then the increment of T, dT = dS + dA So that, Since , After some short evaluation we have, The result follows by taking integration.
An example An ellipse is given by the curve : The area enclosed by the ellipse is therefore : Wow! We get our result easily.