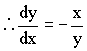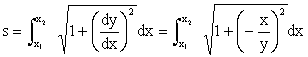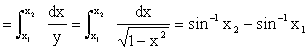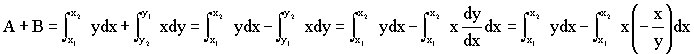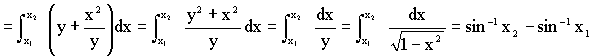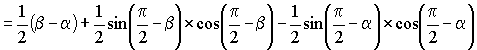A surprise in area and arc-length |
||||||
|
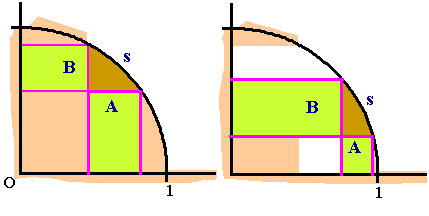
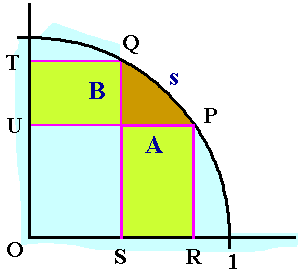
Consider the
first quadrant of a unit circle. Let s be any arc. Then the area between s
and the x-axis plus the area between s and y-axis is a constant! If we forget
about the unit, then A
+ B = s Note that the
regions A and B overlap and that portion of area is counted twice. (in brown) |
|
|||||
|
Let P(x1, y1),
Q(x2, y2) The circle is x2
+ y2 = 1
|
|
|||||
|
\ s = A + B
|
||||||
|
Sector Fun
Let
ÐPOR = a, ÐQOR = b in
radians. Then s = b - a. OP
= OQ = 1 A =
sector POQ + DPOR
- DQOS
(equation
1) |
|
|||||
|
B =
sector POQ + DQOT
- DPOU
Adding
equations 1 and 2, we get: A
+ B = a + b =
s |
||||||
|
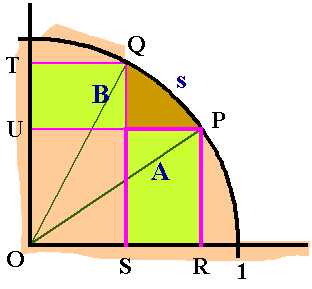
Final
Note : Since the
radius is 1, the two methods above are equivalent because: |
||||||